3.2 Discretization of the demagnetizing field

Another difficulty arises from the calculation of the demagnetizing field. Within each computational cell, the Wigner-Seitz cell of the lattice point, the magnetization is assumed to be homogeneous. We could now try to discretize Poisson's equation () and Laplace's equation (). The main difficulty arises from the open boundary conditions, which are discussed in section . However, for a lattice of homogeneously magnetized cubes, it is possible to calculate the demagnetizing field analytically [23]. The expressions obtained are quite complex and computationally expensive to implement. Since the calculation of the demagnetization field by a magnetic scalar potential is very efficiently implemented in the finite element package, a third possibility has been chosen for the finite difference program. That is the approximation of the demagnetization field of each computational cell by the field of a magnetic dipole in the centre of the cell with the magnetic moment $\mathbf{m}_i = \mathbf{M}_i \Delta x^3$ [24,25].

$$\mathbf{H}_\mathrm{dip}=-\frac{1}{4\pi} \sum_{j \not = i} ... ...3\frac{\mathbf{R}_{ij}(\mathbf{M}_j\mathbf{R}_{ij})}{R_{ij}^5}$$

The inaccuracy is not large and the true long-range nature of the problem is kept [26]. This is due to the fact, that the quadrupole moment of a uniformly magnetized cube is identically zero. Only the next term in a multipole expansion, the octapole term, would give an non-zero contribution [27]

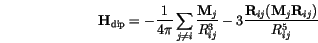
The contributions by the external field and the magnetocrystalline anisotropy to the effective field are straightforward, and they are all summarized in chapter .